School of Mathematics & Statistics

D-modules

 

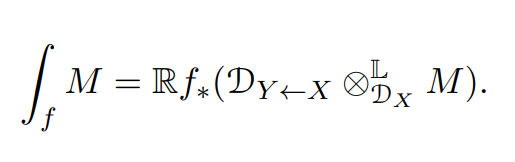
D-modules play a central role in geometric representation theory, acting as a bridge between representation theory and topology via algebraic geometry. They can be studied from the point of view of non-commutative ring theory, algebraic geometry, representation theory or algebraic analysis. At the University of Glasgow, we focus on the applications of D-modules to representation theory, especially in the equivariant setting where D-modules in the presence of a Lie group action can be used to understand the behaviour of invariant eigen-distributions or characters of equivariant sheaves.